
Practice Test: Middle School Mathematics (65)
Suggested Testing Time: 4 hours
To Take This Practice Test
- Use the answer key to record your responses.
- Prefer to take it offline? You can print the questions and answer key.
Remember:
- The practice test can give you a good indication of how you may perform on an actual test, but there is no guarantee that your results will be the same as on the actual test.
- The actual test looks and operates differently than this practice test. In addition, this test includes one or more assignments that allow you to handwrite and scan your responses. Review the Testing Tutorials and Demonstrations for more information about the actual test platform.
- Formulas
 are provided on-screen with your actual test.
are provided on-screen with your actual test.
Question 1.
Given that a is irrational and b is rational, which of the following expressions could result in a rational value?
- a plus b
- a divided by b
- a raised to the power of b
- a minus b
Question 2.
Use the diagram below to answer the question that follows.
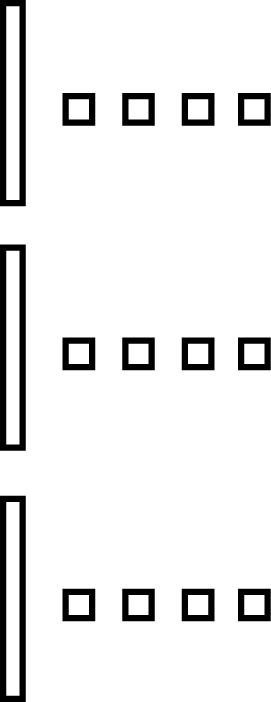
A diagram shows three groups, each containing one rod and 4 unit squares.
Assuming that a rod represents x and a small square represents 1 unit, the algebra tiles shown in the diagram best represent which of the following expressions?
- 3x plus 4
- 3 times the quantity x plus 4
- the cube of the quantity x plus 4
- x cubed plus 4 cubed
Question 3.
If K is both a multiple of x and a factor of y, then which of the following statements must be true?
- k divided by y is a whole number.
- x over k is a whole number.
- y is a factor of x.
- y is a multiple of x.
Question 4.
The greatest common factor of 300, 480, and 840 is 2 to the power of a times 3 to the power of b times 5 to the power of c. What is the value of a plus b plus c?
- 3
- 4
- 5
- 8
Question 5.
Use the multiplication problem below to answer the question that follows.
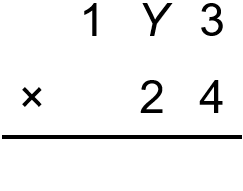
The set-up to multiply the number 1, Y, 3 and 24 using the standard algorithm is shown.
The variable Y represents a single-digit number between 0 and 9. Which of the following expressions represents the amount that Y contributes to the total product?
- 20 times Y
- 24 times Y
- 40 times Y
- 240 times Y
Question 6.
The division problem pen parentheses 2 times 6 to the sixth power plus 10 times 6 to the fourth power plus one half times 6 squared closed parentheses divided by 2 is equivalent to which of the following expressions?
- 2 times 3 to the sixth power plus 10 times 3 to the fourth power plus one half times 3 squared
- 2 times 6 cubed plus 10 times 6 squared plus one half times 6
- 6 cubed plus 5 times 6 squared plus 1 fourth times 6
- 6 to the sixth power plus 5 times 6 to the fourth power plus 1 fourth times 6 squared
Question 7.
Which of the following sets of equations best illustrates the concept that multiplication and division are inverse operations?
-
4 times 1 = 4
4 divided by (negative1) = negative4 -
4 times 0 = 0
0 divided by 4 = 0 -
4 times 8 = 32
32 divided by 8 = 4 -
32 times 1 fourth = 8
32 divided by 8 = 4
Question 8.
A beam of light originating from Saturn travels to Earth with a speed of 3 times ten to the eighth meters per second, and the positions of Saturn and Earth are aligned in diametrically opposite positions about the Sun. Saturn is approximately 1 point 5 times ten to the twelfth meters from the Sun and Earth is approximately 1 point 5 times ten to the eighth meters from the Sun. Which of the following computations provides the best estimate for the number of seconds that elapse until the light becomes visible on Earth?
- 1 third the quantity 1500 plus 0 point 15
- 1 third the quantity 15000 plus 1 point 5
- 1 third the quantity 15000 plus 15
- 1 third the quantity 150000 plus 150
Question 9.
Which of the following values is equivalent to the ratio 2 to the negative third power times 3 all divided by three to the negative second power times 5 squared?
- 3 tenths
- 8 seventy-fifths
- 27 two-hundredths
- 75 seventy-seconds
Question 10.
Use the information below to answer the question that follows.
A teacher uses algebra tiles to demonstrate 3 minus (negative3) to students. The teacher's work is shown below.
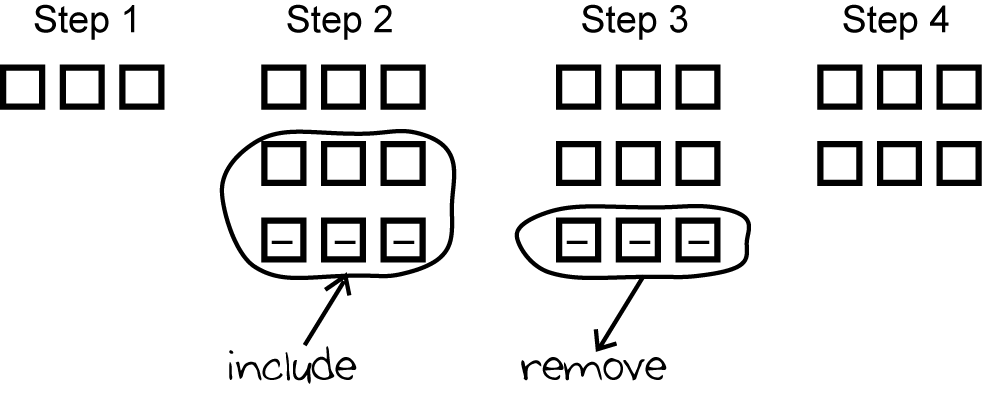
Step 1 shows 3 algebra tiles. Step 2 shows adding a group of 6 tiles, 3 of which have a minus sign on them. Step 3 shows removing the 3 tiles with a minus sign on them. Step 4 shows 6 algebra tiles.
Which of the following properties best justifies Step 2 of the work shown?
- additive identity property
- associative property
- commutative property
- multiplicative inverse property
Question 11.
A submarine is traveling 375 feet below sea level. It rises 183 feet and then dives 228 feet. If the submarine can come safely to the surface at 30 feet per second, approximately how long will it take to reach the surface?
- 11 seconds
- 14 seconds
- 20 seconds
- 26 seconds
Question 12.
An electronics store applies a 15 percent discount on a certain television, which is then followed by a 6 point 5 percent sales tax. If K represents the final amount a customer pays for the television, which of the following expressions can be used to find the original price of the television?
- the quantity 1 minus 0 point 1 5 times 1 point 0 6 5 k
- the quantity 1 plus 0 point 1 5 times 0 point 9 3 5 k
- k divided by the product of 0 point 8 5 and the quantity 1 minus 0 point 0 6 5
- k divided by the product of 0 point 8 5 and the quantity 1 plus 0 point 0 6 5
Question 13.
Which of the following fractions represents the repeating decimal 0 point 0 5 repeating?
- 5 ninths
- 5 nintieths
- 5 ninety-ninths
- 5 nine-hundred ninetieths
Question 14.
Use the fractions below to answer the question that follows.
A over 270 , B over 450 , C over 1250 , D over 3250
Given that the four fractions shown are reduced to lowest terms, which one will have a terminating decimal equivalent?
- A over 270
- B over 450
- C over 1250
- D over 3250
Question 15.
If x and y are whole numbers whose greatest common factor is 3, which of the following expressions is the least common denominator for 2 over x plus 5 over y?
- 10xy
- 3xy
- 1 third x y
- 1 tenth x y
Question 16.
Given that a over b is a proper fraction greater than zero, which of the following inequalities is true for all positive integer values of c?
- the ratio a minus b over b minus c is greater than a over b
- the ratio a plus c over b plus c is greater than a over b
- the ratio a b over b c is greater than a over b
- the ratio a divided by c over b divided by c is greater than a over b
Question 17.
A space probe that is moving at an average speed of 35,960 miles per hour needs to travel approximately 43 point 1 million miles more on its journey to Mars. Which of the following is the best estimate of the number of days it should take the probe to reach Mars?
- 24 days
- 50 days
- 240 days
- 500 days
Question 18.
Coasting down a hill, a bicyclist's speed increases at a constant rate of 0 point 7 5 mile per hour each second. If the bicyclist started timing the ride at the top of the hill when the speed was 5 miles per hour, how many seconds passed before the bicyclist reached a speed of 31 miles per hour?
- 19and 1 half seconds
- 23and 1 fourth seconds
- 34and 2 thirds seconds
- 41and 1 third seconds
Question 19.
The average distance between Earth and the Sun is 149,598,000 kilometers. When Mars and Earth are on the same side of the Sun, the distance between them is approximately 0.5 times the distance from Earth to the Sun. A spacecraft is expected to take approximately 9 months to travel from Earth to Mars. Which of the following intervals is a reasonable estimate for the average speed of a spacecraft traveling from Earth to Mars?
- 100,000 kilometers per hour to 150,000 kilometers per hour
- 10,000 kilometers per hour to 15,000 kilometers per hour
- 1,000 kilometers per hour to 2,000 kilometers per hour
- 100 kilometers per hour to 200 kilometers per hour
Question 20.
Which of the following figures describes mixed number Awholes plus b over c in simplest form such that Abc = 8?
-

Three congruent bars are divided into fifths. The first bar has the first 3 fifths shaded plus the second bar has all fifths shaded plus the last bar has the last 3 fifths shaded.
-
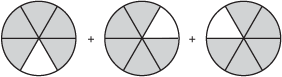
Five congruent circles are divided into fifths. The first circle has 4 fifths shaded plus the second circle has a different set of 4 fifths shaded plus the final circle has a different set of 4 fifths shaded.
-
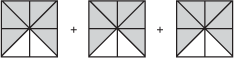
Three congruent squares are divided into eighths. Shown is the sum of the three squares where each square has the same 6 eighths shaded.
-

Four congruent circles are divided into fourths. The first circle has one fourth shaded plus the second circle has 3 fourths shaded plus the third circle has one fourth shaded.
Question 21.
Given that the points on each of the number lines shown below are equally spaced, on which of the following number lines does point D correspond with the fraction 1 fourth?
-
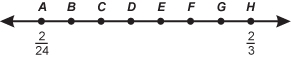
A number line is shown with 8 uniformly spaced points A through H. A is labeled 2 twenty fourths and H is labeled 2 thirds.
-
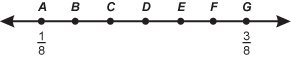
A number line is shown with 7 uniformly spaced points A through G. A is labeled 1 eighth and G is labeled 3 eighths.
-
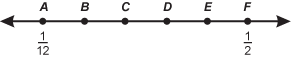
A number line is shown with 6 uniformly spaced points A through F. A is labeled 1 twelfth and F is labeled one half.
-

A number line is shown with 5 uniformly spaced points A through E. A is labeled 1 seventh and E is labeled 3 sevenths
Question 22.
Two rectangular house lots are for sale. Although the width is different for the two lots, they are both 110 feet long. The area of lot A is 4950 feet and the area of lot B is 7920 feet. What is the ratio of the width of lot A to the width of lot B?
- 5 to 8
- 5 to 13
- 8 to 5
- 8 to 13
Question 23.
Use the table below to answer the question that follows.
| Diameter (miles) | 0 | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|---|
| Days | 0 | 4 | 9.5 | 16.5 | 25 | 35 |
The table shows the number of days an offshore oil well has been leaking as a function of the diameter in miles of the oil spill. Assuming that the pattern shown in the table continues, in how many days will the diameter of the oil spill be 8 miles?
- 50 days
- 65 days
- 74 days
- 82 days
Question 24.
Use the sequence below to answer the question that follows.
1, 2, 4, 8, 16, 32, …
Which of the following statements is true about the sequence shown?
- The sum of any two terms in the sequence is also within the sequence.
- The product of any two terms in the sequence is also within the sequence.
- The average of any two terms in the sequence is also within the sequence.
- The quotient of any two terms in the sequence is also within the sequence.
Question 25.
Use the table below to answer the question that follows.
| n | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| value | 2 | negative6 | negative14 | negative22 |
If the sequence of numbers continues using the same pattern, which of the following expressions represents the value of the nth term in the sequence?
- 2 minus 8 n
- 10 minus 8 n
- 8 n minus 2
- 8 n minus 10
Question 26.
A nurse administers 100 milligrams of a medication to a patient at noon. The amount of the medication in the patient's bloodstream decreases at a rate of 10% per hour. Which of the following types of mathematical function best describes the relationship between the number of hours since noon and the amount of medication in the patient's bloodstream at that time?
- exponential
- linear
- proportional
- quadratic
Question 27.
Use the table below to answer the question that follows.
| Celsius | Fahrenheit |
|---|---|
| 10 degrees | 50 degrees |
| 15 degrees | 59 degrees |
| 20 degrees | 68 degrees |
| 25 degrees | 77 degrees |
| 30 degrees | 86 degrees |
The table shows the mathematical relationship between degrees Celsius and degrees Fahrenheit. This mathematical relationship is best described as:
- constant.
- quadratic.
- exponential.
- linear.
Question 28.
A tournament has 256 participants. For each round of the tournament, half of the teams will move on to compete in the next round and half will be eliminated. Which of the following expressions describes the total number of teams that have been eliminated in the tournament after 3 rounds have been completed?
- 2 to the power of 8 plus 2 to the power of 6 plus 2 to the power of 4
- 2 to the power of 8 plus 2 to the power of 7 plus 2 to the power of 6
- 1 half times the quantity 2 to the power of 7 plus 2 to the power of 6 plus 2 to the power of 5
- 1 half times the quantity 2 to the power of 8 plus 2 to the power of 7 plus 2 to the power of 6
Question 29.
Use the table below to answer the question that follows.
| Volume (cubic centimeters) | 20 | 25 | 30 | 36 | 40 | 45 |
|---|---|---|---|---|---|---|
| Pressure (pounds per square inch) | 180 | 144 | 120 | 100 | 90 | 80 |
The table shows the relationship between the volume in cubic centimeters of a particular gas and its pressure in pounds per square inch at a constant temperature. The relationship between volume and pressure can best be defined as:
- exponential.
- inversely proportional.
- linear.
- quadratic.
Question 30.
Use the diagram below to answer the question that follows.

A pattern is shown with a triangular arrangement of dots composed of three dots on the bottom, two dots above those, and one dot at the top. For each iteration of the pattern, a larger triangle is formed by adding another row at the bottom that contains one more dot than the bottom row of the previous triangle.
In the diagram above, which of the following equations represents the relationship between the number of dots, d, in a triangle and the number of rows, R, in that triangle?
- d equals 2 times the quantity R plus 3 all divided by 2
- d equals R times the quantity R plus 1 all divided by 2
- d equals 2 times the quantity R plus 6 all divided by 3
- d equals 2 times the quantity R squared all divided by 3
Question 31.
Use the diagram below to answer the question that follows.
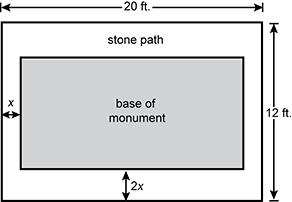
The diagram shows a rectangle surrounded by a stone path, where x represents the narrower path-width along the short sides of the rectangle and 2x represents the wider path width along the long sides of the rectangle. The region containing the stone path and monument is a 20-foot by 12-foot rectangle.
The diagram shows how the rectangular base of a monument is surrounded by a stone path, where x represents the narrower path-width. The width of the stone path on the longer side of the monument is twice the width on the shorter side. If the region containing the stone path and monument is a 20-foot by 12-foot rectangle, which of the following expressions represents the area of the base of the monument in square feet?
- 2 times the trinomial x squared minus 22 x plus 120
- 2 times the trinomial x squared minus 26 x plus 120
- 8 times the trinomial x squared minus 11 x plus 30
- 8 times the trinomial x squared minus 13 x plus 30
Question 32.
Which of the following equations can be used to determine x, the number of liters of 15 percent acid solution that must be added to 3 liters of a 3 percent acid solution to obtain a 12 percent acid solution?
- 0 point 0 9 x plus 0 point 1 5 equals 0 point 1 2 x
- 0 point 1 5 x plus 0 point 0 9 equals 0 point 1 2 x plus 3
- 0 point 1 5 x plus 0 point 0 9 equals 0 point 1 2 x plus 0 point 3 6
- 0 point 0 9 x plus 0 point 1 5 equals 0 point 1 2 x plus 0 point 3 6
Question 33.
What is the value of 2 times x to the negative second power minus 4 times x to the negative first power when x = 2 fifths?
- 0
- 5 halves
- 15 sixteenths
- 32 twenty-fifths
Question 34.
Which of the following graphs displays the solution set for the inequality negative 2 thirds times the quantity 3 m minus 2 is less than one half times the quantity m plus 1?
-
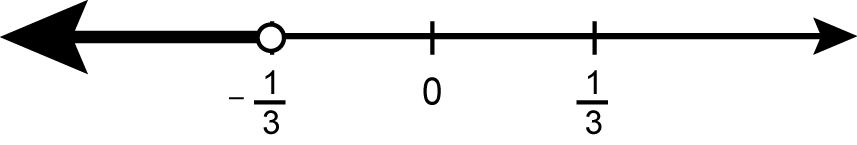
A number line is shown with an open circle on negative one third and a shaded line extending to the left.
-
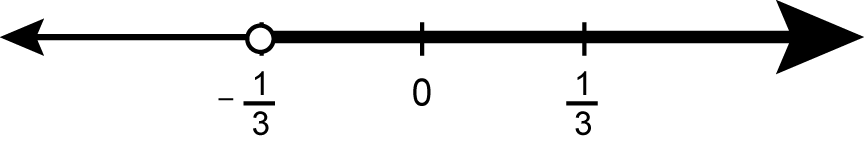
A number line is shown with an open circle on negative one third and a shaded line extending to the right.
-
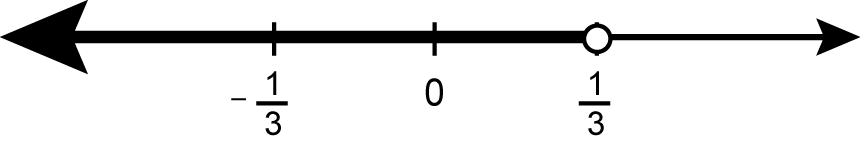
A number line is shown with an open circle on one third and a shaded line extending to the left.
-
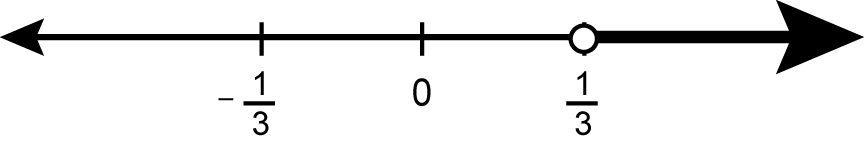
A number line is shown with an open circle on one third and a shaded line extending to the right.
Question 35.
Which of the following expressions represents the value of the binomial a plus c raised to the power of b, all divided by b times the quantity a squared minus c squared when a = 5, b = 4, and c = negative2?
- negative 1 twenty-eighths
- 1 half
- 81 one-hundred sixteenths
- 27 twenty-eighths
Question 36.
Use the graphs below to answer the question that follows.
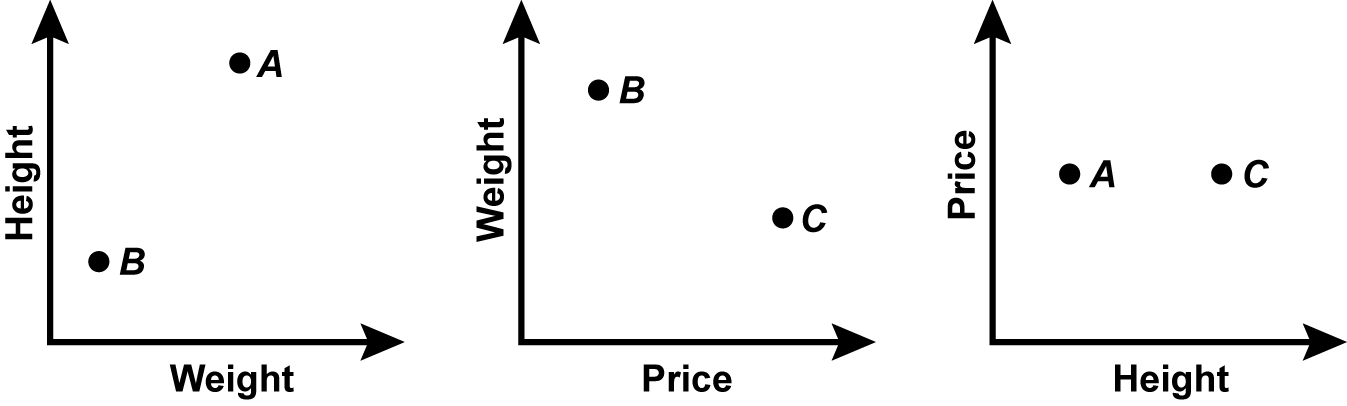
Three graphs are shown. The first graph has vertical axis label "height" and horizontal axis label "weight", with point B at approximately 1, 1 and point A at approximately 4, 5. The second graph shows a vertical axis label of weight and a horizontal axis label of price, with point B at approximately 2, 5, and point C at approximately 5, 2. The third graph shows a vertical axis label of price and a horizontal axis label of height, with Point A at approximately 2 3 and Point C at approximately 4 3.
The height, weight, and price of 3 soup cans, A, B, and C, are compared and the results are shown in the graphs. Based on the information in the graphs, which of the following statements is true?
- Can C weighs the least.
- Cans A and B are the same height.
- Can B is the most expensive.
- Can A is the tallest.
Question 37.
Use the information below to answer the question that follows.
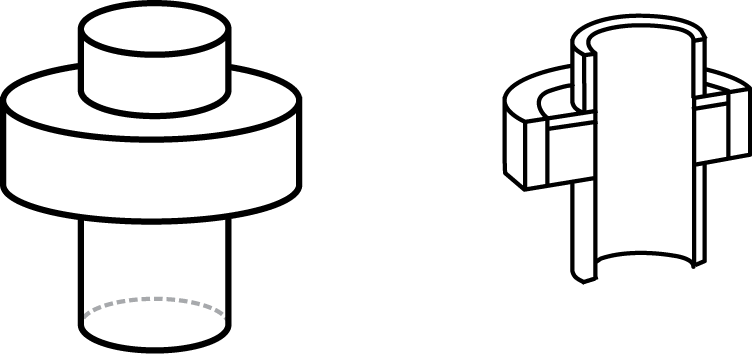
An open top container is shown that is composed, from bottom to top, of a tall cylinder with radius r, a shorter cylinder with radius 2r, and then an even shorter cylinder with radius r.
A container is made up of different cylindrical sections as shown in the diagram. Water is poured into the container at a constant rate. Which of the following graphs best shows the relationship between time and height of water in the container?
-
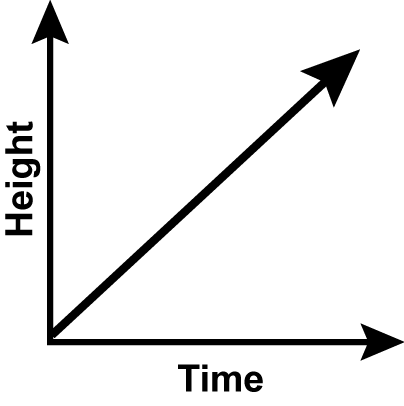
The first quadrant of a coordinate plane is shown with a vertical axis labeled "height" and a horizontal axis labeled "Time". The graph shows a line passing through the origin with a positive rate of change.
-
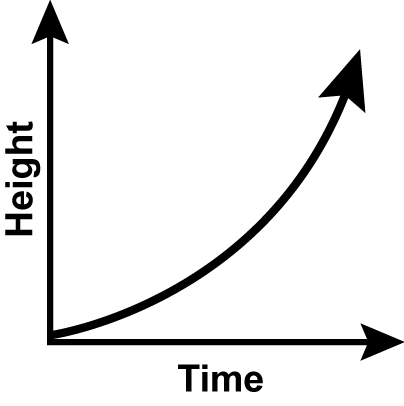
The first quadrant of a coordinate plane is shown with a vertical axis labeled "height" and a horizontal axis labeled "Time". The graph shows a curved line, similar to the right half of a parabola with a vertex at the origin.
-
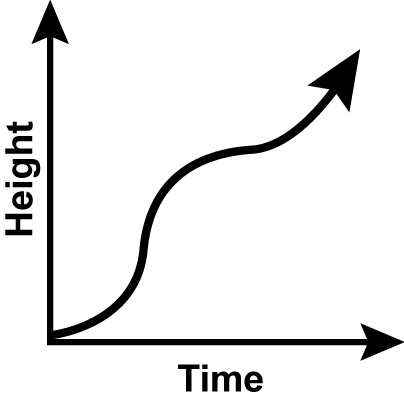
The first quadrant of a coordinate plane is shown with a vertical axis labeled "height" and a horizontal axis labeled "Time". The graph shows a curvy wave pattern with a midline of a diagonal through the origin.
-
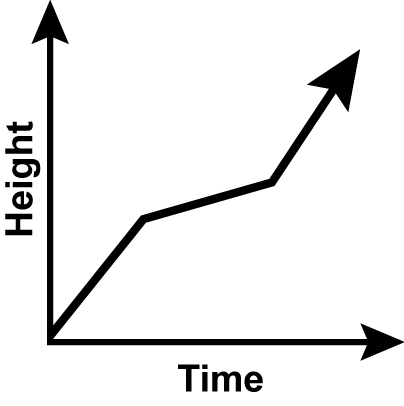
The first quadrant of a coordinate plane is shown with a vertical axis labeled "height" and a horizontal axis labeled "Time". The graph shows a line with a steep positive rate of change, then a lesser positive rate of change, followed by the initial rate of change.
Question 38.
Given that f of x = x squared and g of x = the quantity x plus 5 squared, which of the following statements describes the relationship between f of x and g of x?
- The graph of g of x is equivalent to the graph of f of x minus 5.
- Shifting the graph of g of x to the left 5 units results in the graph of f of x.
- The graph of f of x is equivalent to the graph of g of x minus 5.
- Shifting the graph of f of x to the left 5 units results in the graph of g of x.
Question 39.
Which of the following graphs represents a function?
-
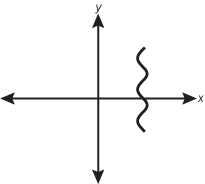
A wavy vertical line is shown in the coordinate plane.
-
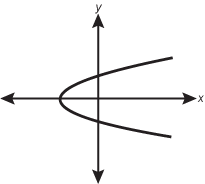
A parabola that opens to the right is shown in the coordinate plane.
-
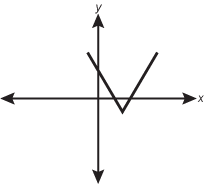
A graph in the shape of the letter V is graphed in the coordinate plane.
-
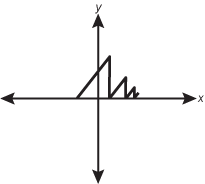
A wave is shown with vertical line segments connecting progressively smaller line segments with the same positive slope.
Question 40.
The first part of a long car trip is made at highway speed. The driver then reduces the speed of the car in traffic, later resuming highway speed before exiting the highway and following local roads to the destination. The return home is at a steady rate on local roads. Which of the following graphs represents the relationship of distance from home, d, to time, t, for the round trip?
-
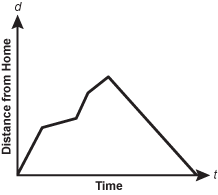
The first quadrant of a coordinate plane is shown with "Distance from Home, d" on the vertical axis and "Time, t" on the horizontal axis. The graph shows a line with the following characteristics, starting at the origin: a steep positive slope, then a lesser positive slope, then the initial slope, then a lesser positive slope, then a negative slope to the t axis.
-
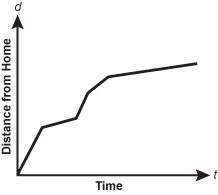
The first quadrant of a coordinate plane is shown with "Distance from Home, d" on the vertical axis and "Time, t" on the horizontal axis. The graph shows a line with the following characteristics, starting at the origin: a steep positive slope, then a lesser positive slope, then the initial slope, then a lesser positive slope, then an even lesser positive slope.
-
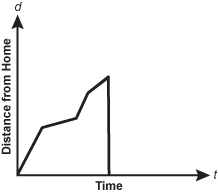
The first quadrant of a coordinate plane is shown with "Distance from Home, d" on the vertical axis and "Time, t" on the horizontal axis. The graph shows a line with the following characteristics, starting at the origin: a steep positive slope, then a lesser positive slope, then the initial slope, then a lesser positive slope, then a vertical line to the t axis.
-
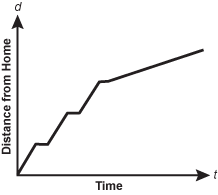
The first quadrant of a coordinate plane is shown with "Distance from Home, d" on the vertical axis and "Time, t" on the horizontal axis. The graph shows a line with the following characteristics, starting at the origin: a steep positive slope, then a zero slope, then the initial slope, then a zero slope, then the initial slope, then a zero slope, followed by a lesser positive slope.
Question 41.
Given that f of x represents a direct variation function with constant of variation negative 2 thirds, and (x, y) is any point on the graph of f of x, which of the following coordinate pairs represents another point on the graph of f of x?
- negative 2 x, 2 y
- 2 x, 2 y
- negative 2 x, 3 y
- 2 x, 3 y
Question 42.
A local school requires 3 teachers as chaperones for every 20 students attending a field trip. Which of the following graphs best relates x, the number of students, to y, the number of teachers, on any given field trip?
-
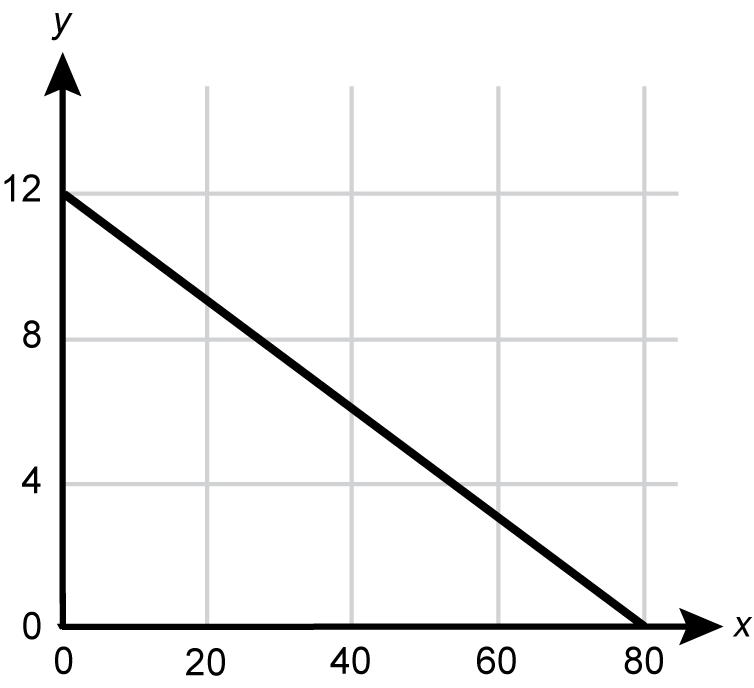
A graph of the line is shown in the first quadrant of the coordinate plane. The line has a y intercept of 12 and an x intercept of 80.
-
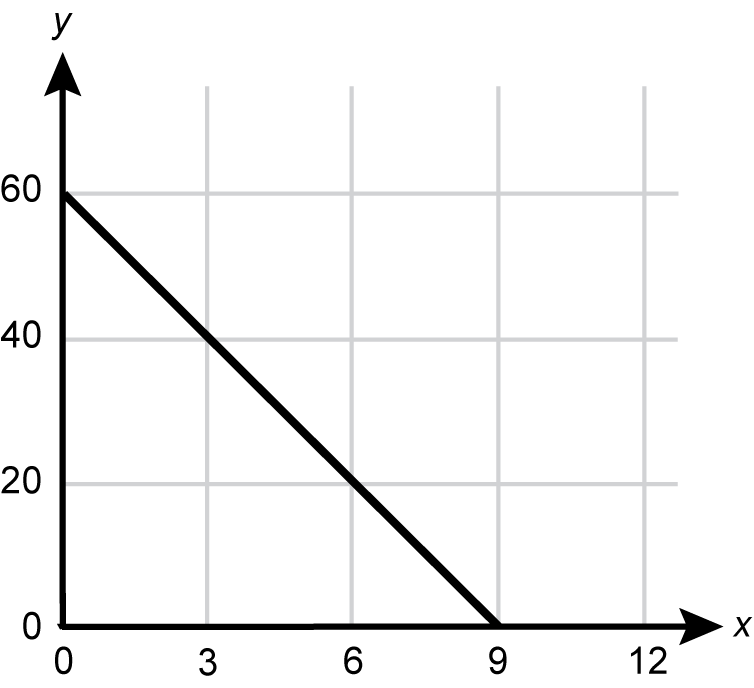
A graph of the line is shown in the first quadrant of the coordinate plane. The line has a y intercept of 60 and an x intercept of 9.
-
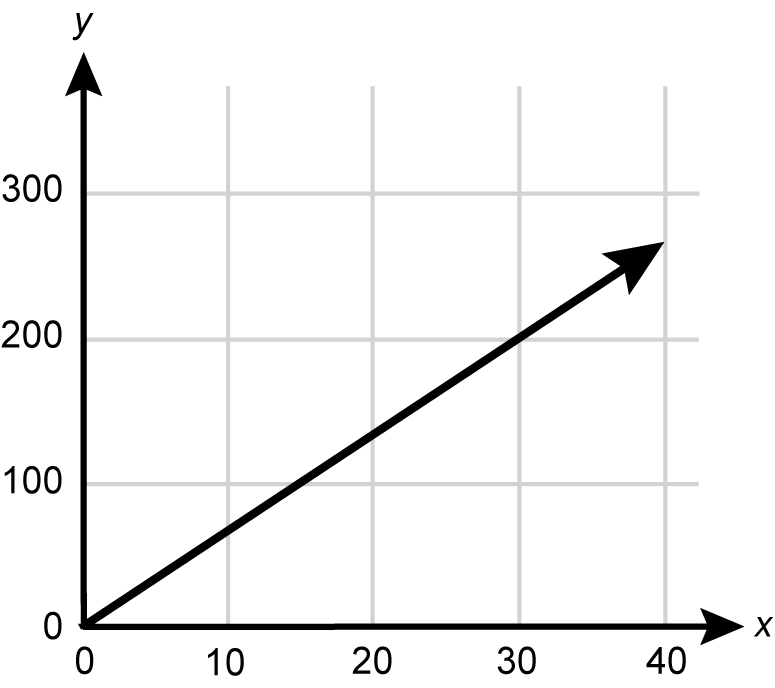
A graph of the line is shown in the first quadrant of the coordinate plane. The line has passes through the origin and the point 30, 200.
-
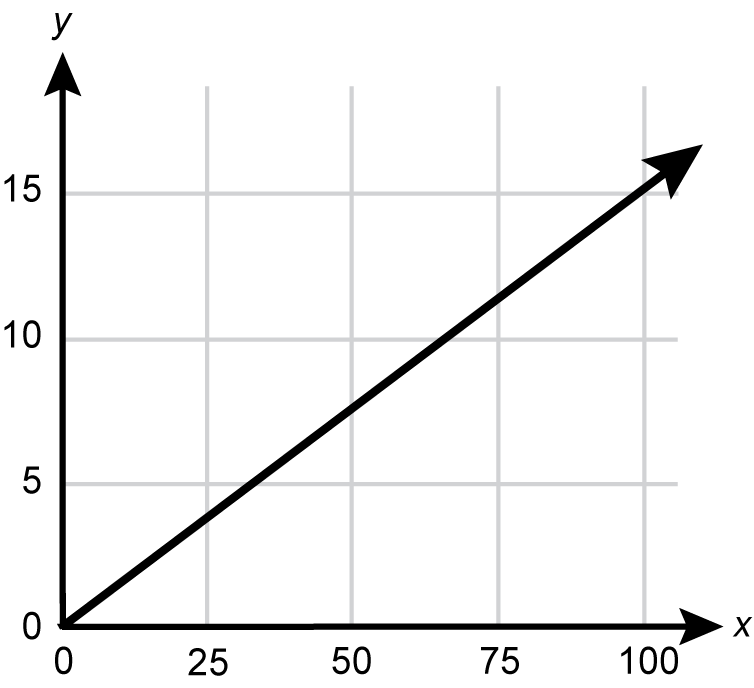
A graph of the line is shown in the first quadrant of the coordinate plane. The line passes through the origin and the point 100, 15.
Question 43.
Two points, negative 2, k plus 8 and 2, k, lie on line L. Which of the following expressions represents the x-intercept of line L in terms of k?
- k plus 4
- negative k minus 4
- 1 half the quantity k plus 4
- 1 half the quantity k minus 4
Question 44.
Two lines, k and p, are parallel. If the equation for line k is 3 x plus A y equals B, and line p passes through the point negative 4, 0, which of the following equations represents line p?
- y equals 3 over A times the quantity x minus 4
- y equals 3 x divided by A all minus 4
- y equals negative 3 over A times the quantity x plus 4
- y equals negative 3 x divided by A all plus 4
Question 45.
Which of the following graphs shows the solution to the system of inequalities negative 2 times the quantity x minus 3 is less than y plus 7 and 3 x minus 4 y is greater than or equal to 12?
-
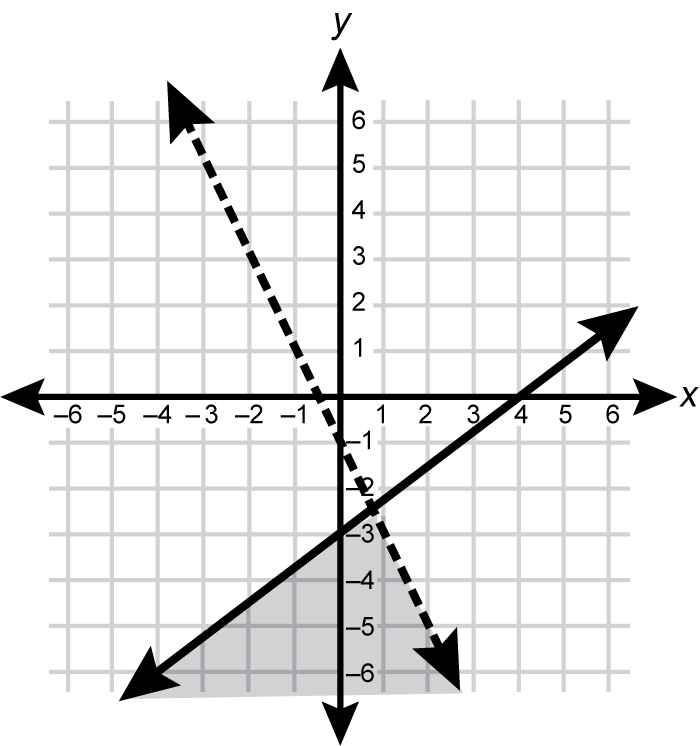
The solid line 3 x minus 4 y = 12 intersects the dotted line y = negative one half x minus 1 to divide the coordinate plane into four regions. The bottom region is shaded.
-
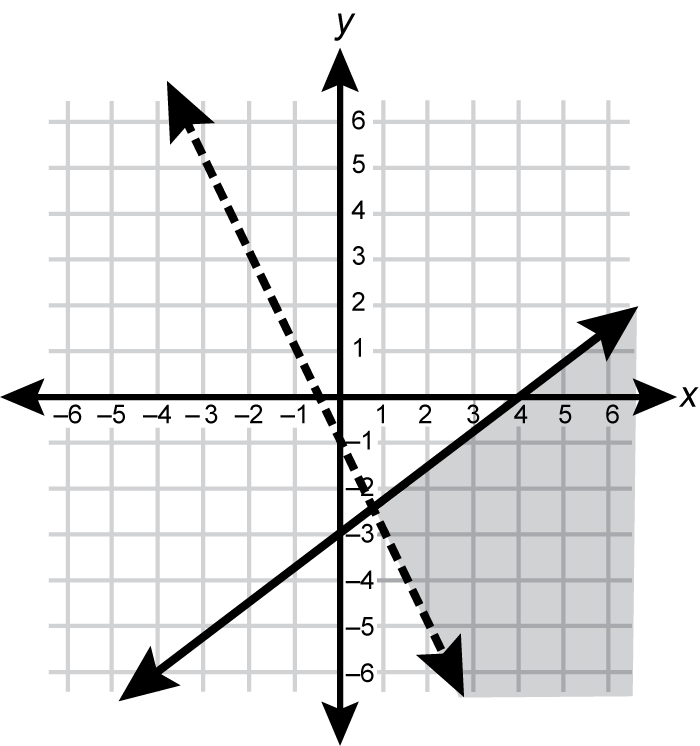
The solid line 3 x minus 4 y = 12 intersects the dotted line y = negative one half x minus 1 to divide the coordinate plane into four regions. The rightmost region is shaded.
-
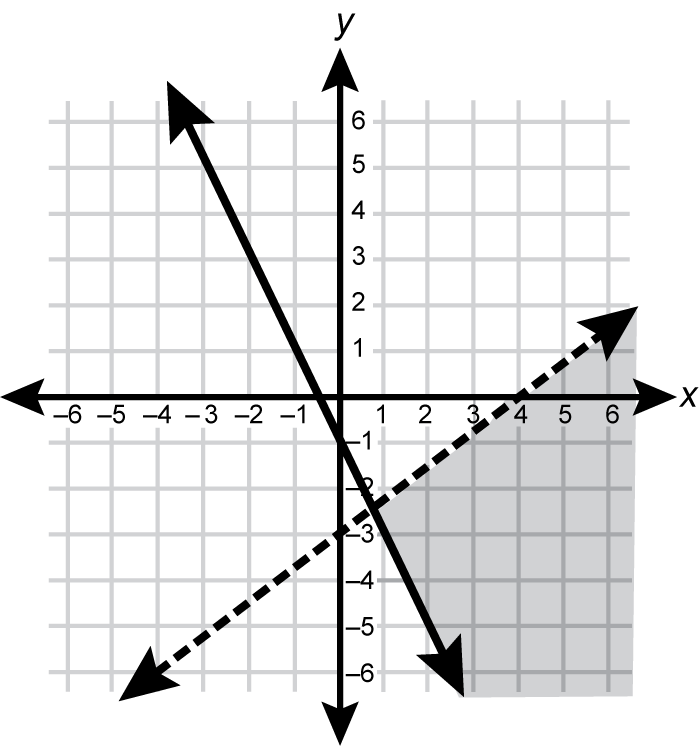
The dotted line 3 x minus 4 y = 12 intersects the solid line y = negative one half x minus 1 to divide the coordinate plane into four regions. The rightmost region is shaded.
-
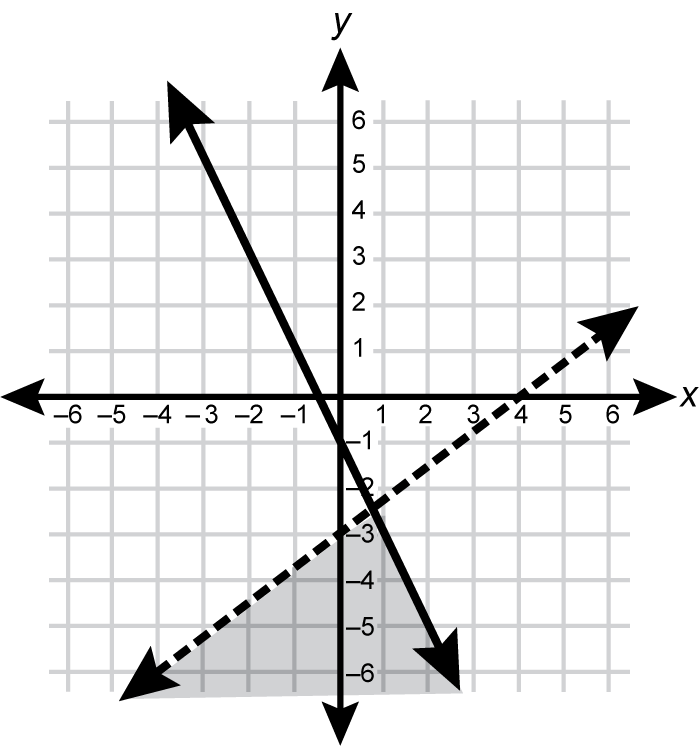
The dotted line 3 x minus 4 y = 12 intersects the solid line y = negative one half x minus 1 to divide the coordinate plane into four regions. The bottom region is shaded.
Question 46.
If 3 x plus 4 y equals negative 20 and 2 x minus 3 y equals negative 2, then what is the value of x?
- x = negative 4
- x = negative 2
- x = negative 28 fifths
- x = negative 22 seventeenths
Question 47.
A teacher has 2-inch-thick textbooks weighing 6 pounds each and 2.5-inch-thick lab manuals weighing 5 pounds each. If the classroom contains a 3-foot-long shelf that can hold up to 85 pounds, which of the following systems of equations represents the number of textbooks, x, and lab manuals, y, that can be placed on the shelf?
-
6 x plus 5 y is less than 85
and 2 x plus 2 point 5 y is less than 36 -
one sixth x plus one fifth y is less than 85
and one half x plus y divided by 2 point 5 is less than 36 -
6 x plus 5 y is less than or equal to 85
2 x plus 2 point 5 y is less than or equal to 36 -
one sixth x plus one fifth y is less than or equal to than 85
and one half x plus y divided by 2 point 5 is less than or equal to 36
Question 48.
Use the graph below to answer the question that follows.
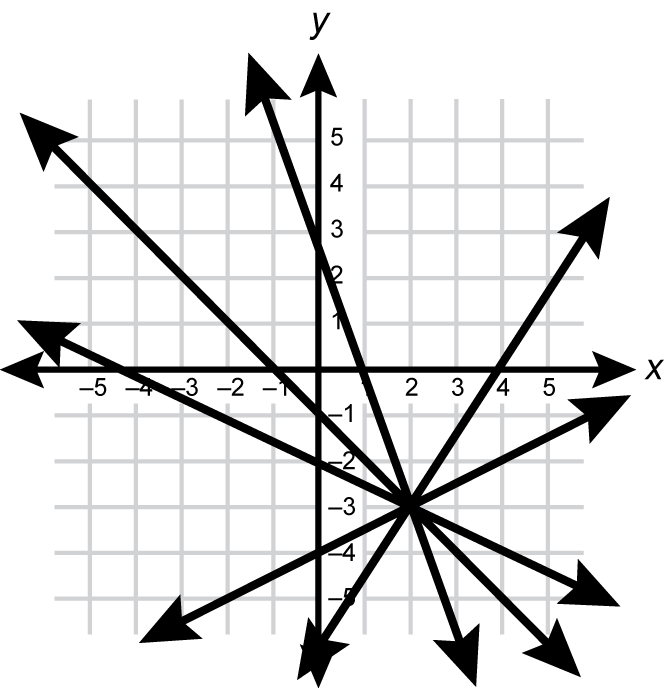
A coordinate plane is shown. A series of lines intersect through the point 2 negative 3 on the graph.
Given that k is a slope value, the family of lines shown in the graph can be described using which of the following equations?
- y plus 3 equals k x minus 2
- y plus 3 equals k times the quantity x minus 2
- y equals k x plus 2 minus 3
- y equals k times the quantity x plus 2 all minus 3
Question 49.
Use the table below to answer the question that follows.
| x | y |
|---|---|
| 1 | 3and 1 third |
| 2 | 2and 2 thirds |
| 3 | 2 |
Which of the following equations represents the function described by the points in the table?
- x minus 3 y equals 6
- 3 y minus x equals 6
- 2 x plus 3 y equals 12
- 2 y plus 3 x equals 12
Question 50.
A particular vehicle loses 15 percent of its value each year. Which of the following expressions represents the value of this vehicle 3 years ago, given that the vehicle is currently valued at 27500 dollars?
- 27500 times the cube of the sum 1 plus 0 point 1 5
- 27500 times the cube of the difference 1 minus 0 point 1 5
- 27500 divided by the cube of the sum 1 plus 0 point 1 5
- 27500 divided by the cube of the difference 1 minus 0 point 1 5
Question 51.
Use the graph below to answer the question that follows.
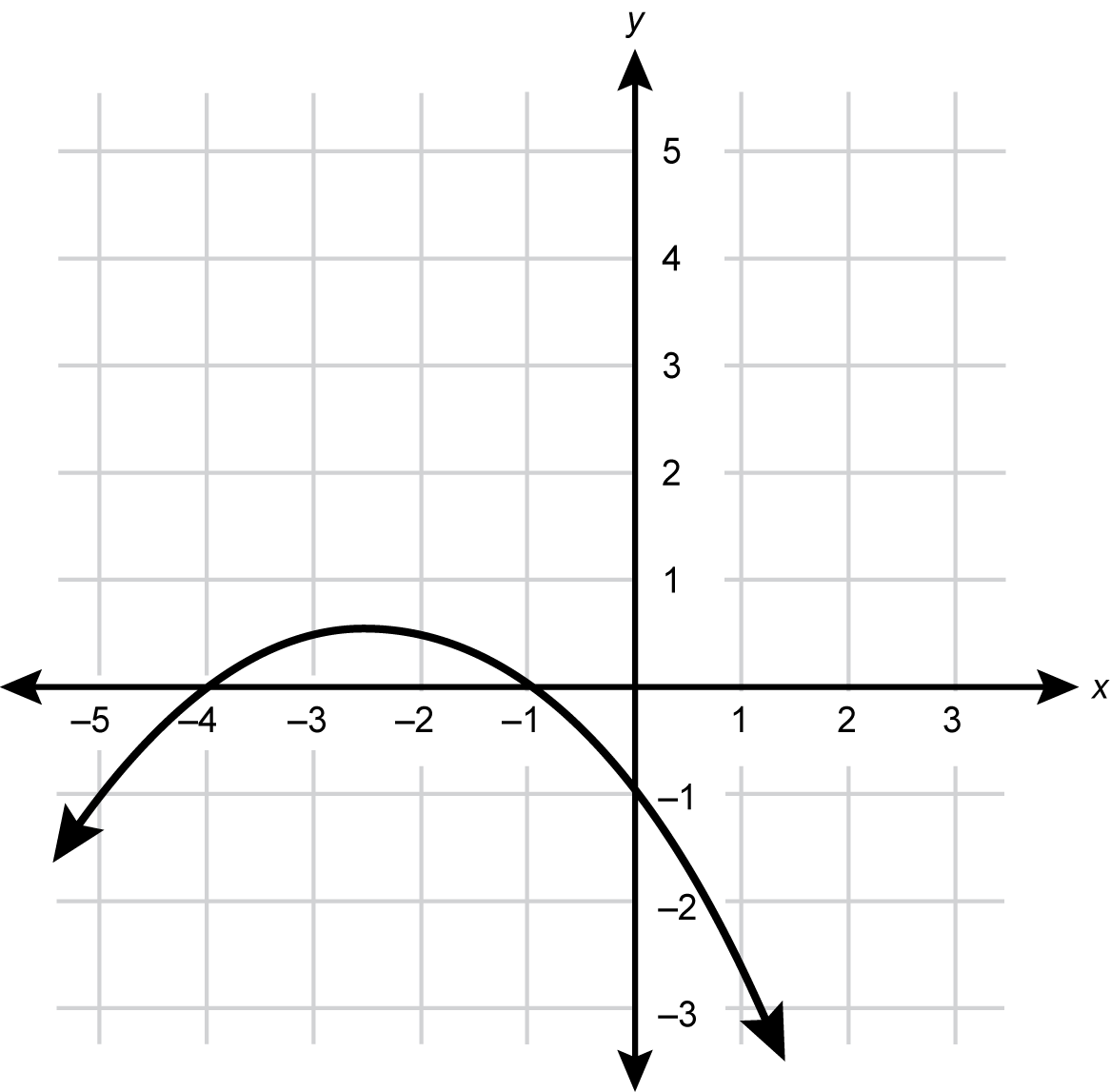
The graph of a parabola that opens down has x intercepts at negative 1 and negative 4 and a y intercept at negative 1.
When the equation of the parabola shown is written in the form y equals a times the quantity x plus b times the quantity x plus c, what is the value of a?
- negative3
- negative1
- negative 1 fourth
- negative 1 half
Question 52.
A farmer triples the area of a square chicken pen by increasing its length by 4 meters and its width by 2 meters. Which of the following equations could be a step in the process of finding x, the side length of the original pen?
- x squared minus 3 x minus 4 equals 0
- x squared plus 6 x plus 8 equals 0
- 2 x squared plus 6 x minus 8 equals 0
- 3 x squared minus 2 x minus 4 equals 0
Question 53.
The graph of a parabola of the form y equals x squared plus b x plus c crosses the y-axis at negative36 and the x-axis at x = 3. At what other point does the graph cross the x-axis?
- x = negative12
- x = negative9
- x = 9
- x = 12
Question 54.
Use the diagram below to answer the question that follows.
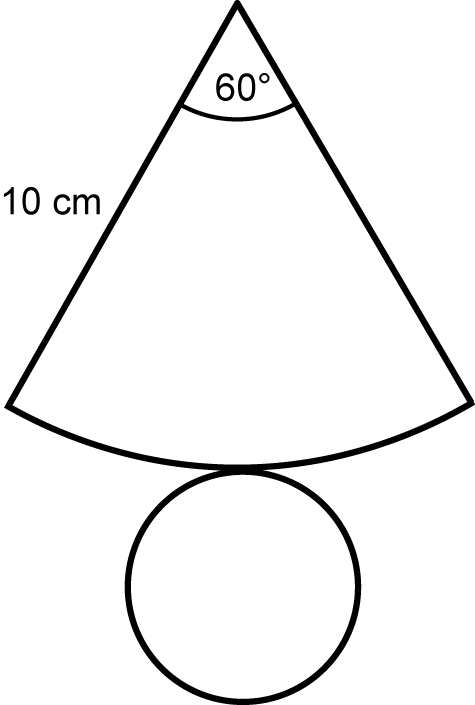
A net for a right cone is shown that depicts a sector for a large circle connected to a smaller circle. The sector for the large circle has a central angle of 60 degrees and a radius of 10 centimeters.
The diagram shows the net of a right cone. Which of the following expressions represents the area of the cone's base in square centimeters?
- 25 pi over 3
- 25 pi over 6
- 25 pi over 9
- 25 pi over 36
Question 55.
The edges of a small cube are scaled by a factor of 4. How does the surface area–to-volume ratio for the larger cube compare to that of the smaller cube?
- The ratio is smaller by a factor of 4.
- The ratio is smaller by a factor of 2.
- The ratio is larger by a factor of 2.
- The ratio is larger by a factor of 4.
Question 56.
The edge of a cube measures 10 inches, rounded to the nearest inch. Using the rounded length, a student calculates the volume of the cube to be 1000 cubic inches. Which of the following computations is most relevant to establishing the maximum amount of error in the student's reported volume?
- the cube of the difference 10 point 4 9 minus 10
- the cube of the difference 10 point 4 9 minus 9 point 5
- 10 cubed minus 9 cubed
- 10 point 4 9 cubed minus 10 cubed
Question 57.
Use the diagram below to answer the question that follows.
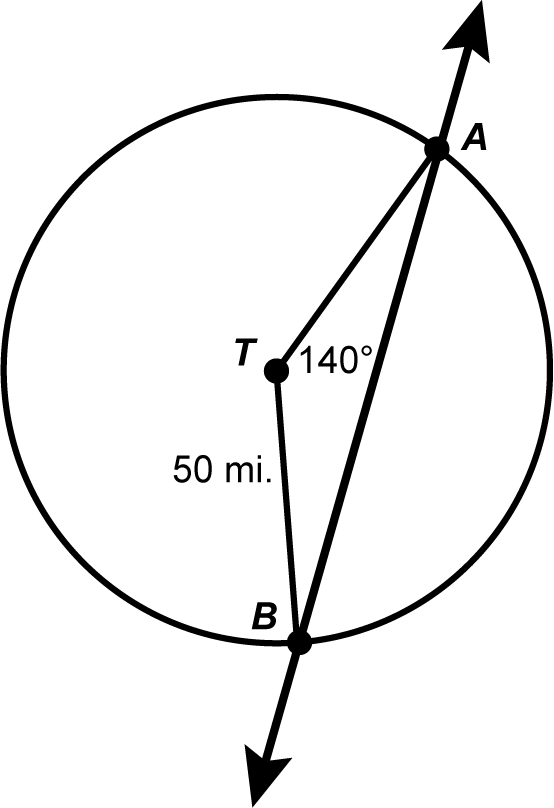
Circle T has a chord A B that is not a diameter. Isosceles triangle T A B is formed with central angle T measuring 140 degrees. The length of TB is 50 miles.
The signal from cell tower T extends over a circular area for a radius of 50 miles with highway A B passing through the service area as shown. If angle A T B = 140 degrees, which of the following expressions represents the length, in miles, of highway A B within the service area?
- 50 sine of 140 degrees
- 100 sine of 70 degrees
- 50 cosine of 140 degrees
- 100 cosine of 70 degrees
Question 58.
Which of the following expressions is equal to the area of an isosceles triangle with a base angle θ, a base of length 16, and legs of length 12?
- 48 cosine theta
- 96 cosine theta
- 48 sine theta
- 96 sine theta
Question 59.
Use the diagram below to answer the question that follows.
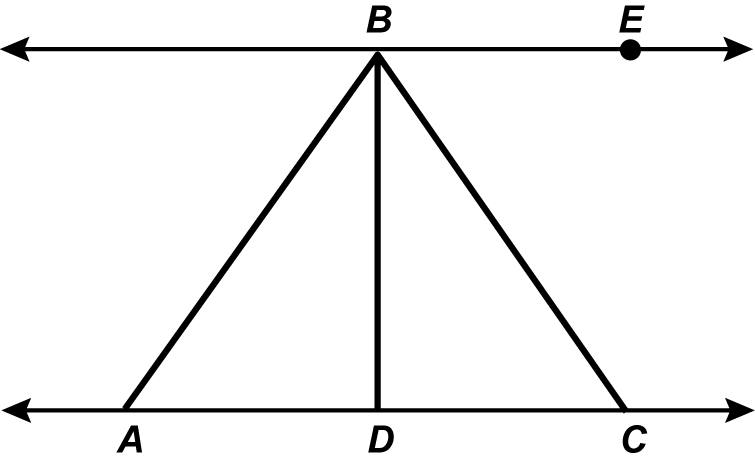
Two horizontal lines are shown. Triangle A B C is drawn between them such that vertex B is on the top line and vertices A and C are on the bottom line. There is a line segment B D that connects vertex B to a point D that is between A and C on the bottom line. There is also point E on the top line, to the right of vertex B.
Which of the following assumptions can be made about the figure shown?
-
Point A is on
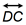
- line A C is congruent to line B C
- angle A D B is a right angle
-
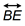 is parallel to
is parallel to 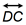
Question 60.
Use the diagram below to answer the question that follows.
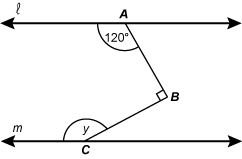
Two horizontal parallel lines l and m are shown. Point B is positioned in the interior of the parallel lines and connected to each line by a line segment such that angle B is a right angle. Segment B A, which connects point B to point A on line L, forms an angle of 120 degrees with line l. Segment B C, which connects point B to point C on line m, forms an angle of y degrees with line m.
Given that line L is parallel to line m, what is the measure of angle y?
- 120 degrees
- 135 degrees
- 150 degrees
- 165 degrees
Question 61.
Triangle A B C is a right triangle with hypotenuse A B. Segment M N is drawn parallel to side A C with M on hypotenuse A B and N on side C B. If A C = 20, C N = 12, and M N = 12, what is the length of side C B?
- 16
- 18
- 20
- 30
Question 62.
Use the diagram and information below to answer the question that follows.
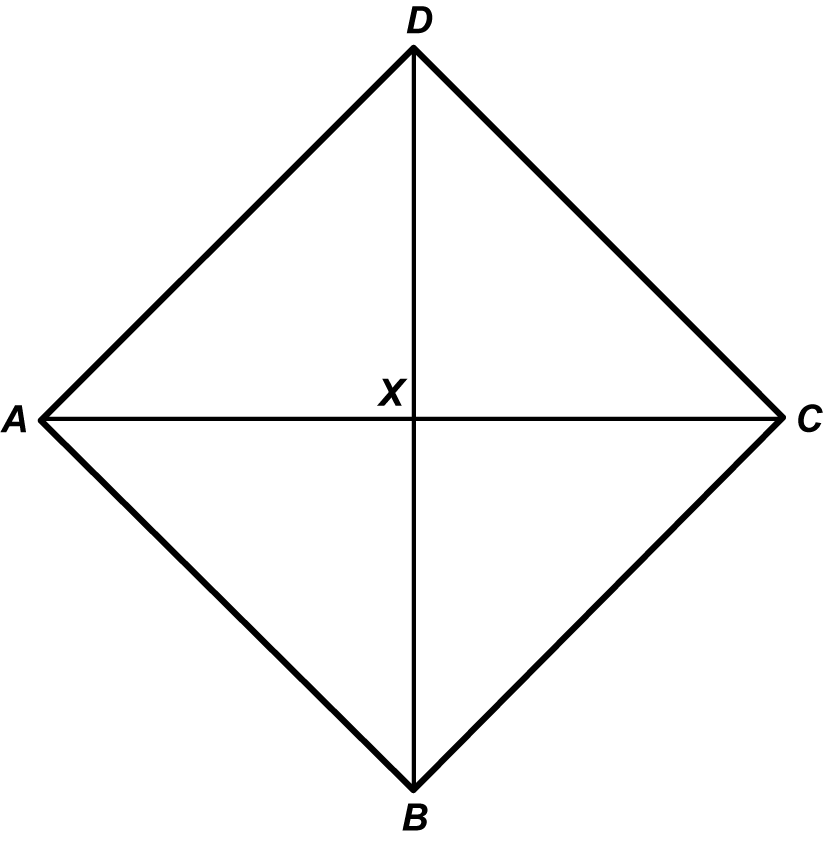
Quadrilateral A B C D is shown with diagonals A C and B D. The intersection of the diagonals forms an angle of x degrees.
If segment A D is congruent to segment A B and segment D C is congruent to segment B C, then which of the following statements must be true?
- segment D X is congruent to segment B X
- segment A X is congruent to segment C X
- angle D A X is congruent to angle A D X
- angle D B C is congruent to angle B D A
Question 63.
Use the diagram below to answer the question that follows.
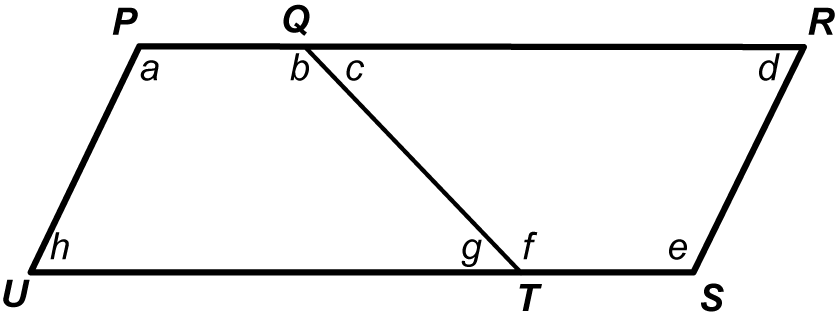
Quadrilateral R S U P is shown with point Q on P R and point T on U S. A line segment connects Q and T which divides quadrilateral R S U P into two quadrilaterals, P Q T U and Q T S R. Quadrilateral P Q T U has interior angle measures of a, b , g, and h respectively. Quadrilateral Q R S T has interior angle measures of c d e and f respectively.
Trapezoid P Q T U is congruent to trapezoid S T Q R. After showing that P Q R and U T S are line segments, which of the following observations best justifies that segment P U and segment R S are parallel?
- P U = R S
- the measure of angle d = the measure of angle h and the measure of angle a = the measure of angle e = 180 degrees minus the measure of angle d
- P Q plus Q R = U T plus T S
- the measure of angle c = the measure of angle g and the measure of angle b = the measure of angle f = 180 degrees minus the measure of angle c
Question 64.
Use the diagram below to answer the question that follows.
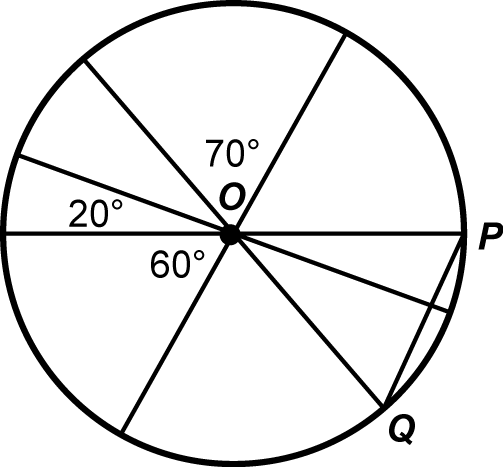
Circle O is shown with 4 diameters and chord P Q such that P Q O forms an isosceles triangle. One diameter is parallel to chord P Q. The central angles formed along this diameter on the side of the circle opposite chord P Q measure 60 degrees, 20 degrees, x degrees, and 70 degrees.
The diagram shows circle O with four diameters and chord P Q. What is the measure of angle O P Q?
- 55 degrees
- 60 degrees
- 65 degrees
- 70 degrees
Question 65.
Use the diagram below to answer the question that follows.
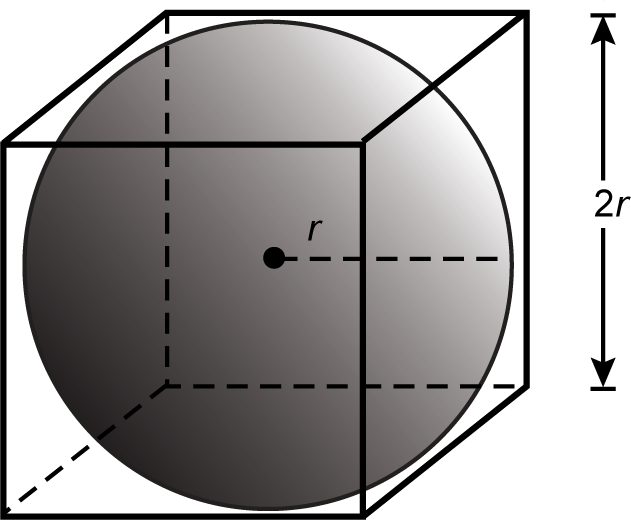
A diagram shows a sphere inscribed in a cube. The radius of the sphere is r, and the side lengths for the cube are 2r.
A sphere is inscribed in a cube as shown in the diagram above. If the volume of the cube is 64 cubic inches, what is the approximate volume of the sphere?
- 19 cubic inches
- 33 cubic inches
- 41 cubic inches
- 50 cubic inches
Question 66.
Use the diagram below to answer the question that follows.
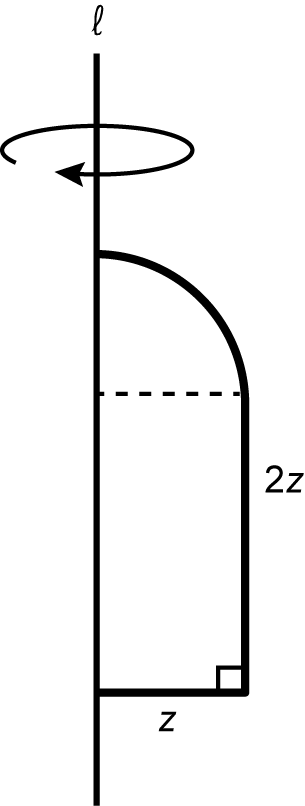
A vertical line, L, is the axis of revolution for a composite shape that is formed by a rectangle connected to a quarter of a circle. The rectangle has dimensions z and 2z, and the radius of the quarter circle is equal to the length of the shared side with the rectangle, z.
The diagram shows a figure made up of a quarter of a circle adjacent to a rectangle that has dimensions z times 2 z. If the figure is rotated about line L, which of the following expressions represents the volume of the rotated figure?
- 7 pi z cubed over 3
- 8 pi z cubed over 3
- 10 pi z cubed over 3
- 14 pi z cubed over 3
Question 67.
Use the diagram below to answer the question that follows.
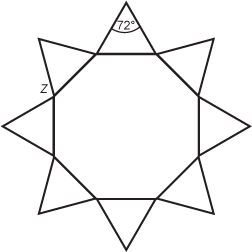
A figure is shown with an octagon at the center. On the outside of the octagon, each of the eight sides forms the base of an isosceles triangle. The angle opposite the base in each triangle is equal to 72 degrees. Angle Z is indicated as the angle formed between two consecutive isosceles triangles on the outside of the octagon.
The diagram above was created by adding isosceles triangles to the sides of a regular octagon. What is the measure of angle Z?
- 135 degrees
- 126 degrees
- 117 degrees
- 108 degrees
Question 68.
A circle with a circumference of 16 pi is constructed on a coordinate grid. The center of the circle is located at 5, 5. Which of the following coordinates could be a point on the circle?
- 5, negative 3
- 5, 1
- negative 3, negative 3
- 21, 5
Question 69.
On the coordinate plane, the bases of an isosceles trapezoid are parallel to the y-axis. If three of its vertices are negative 1, 7, 5, 9, and 5, negative 1, what is its area?
- 42 square units
- 48 square units
- 56 square units
- 64 square units
Question 70.
Points A2, a, B5, b, and C9, c are plotted on a coordinate grid to form angle A B C. If angle A B C is a right angle, which of the following relationships must be true?
- the ratio a minus b over 2 minus 5 is equal to the ratio 5 minus 9 over c minus b
- the ratio a minus b over 2 minus 5 is equal to the ratio 9 minus 2 over a minus c
- the ratio a minus c over 2 minus 9 is equal to the ratio 5 minus 9 over c minus b
- the ratio a minus c over 2 minus 9 is equal to the ratio 9 minus 5 over c minus b
Question 71.
Use the graph below to answer the question that follows.
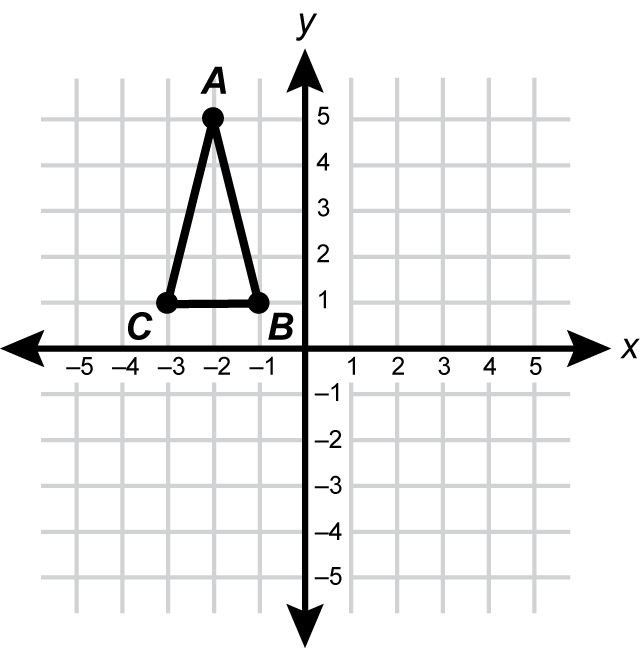
Triangle A B C is graphed in the coordinate plane with vertex A located at negative 2 5, vertex B located and negative 1 1 and vertex C located at negative 3 1.
triangle A B C is reflected over y = x and then rotated 180 degrees counterclockwise about the origin to create triangle A prime B prime C prime. Which of the following graphs represents triangle A prime B prime C prime?
-
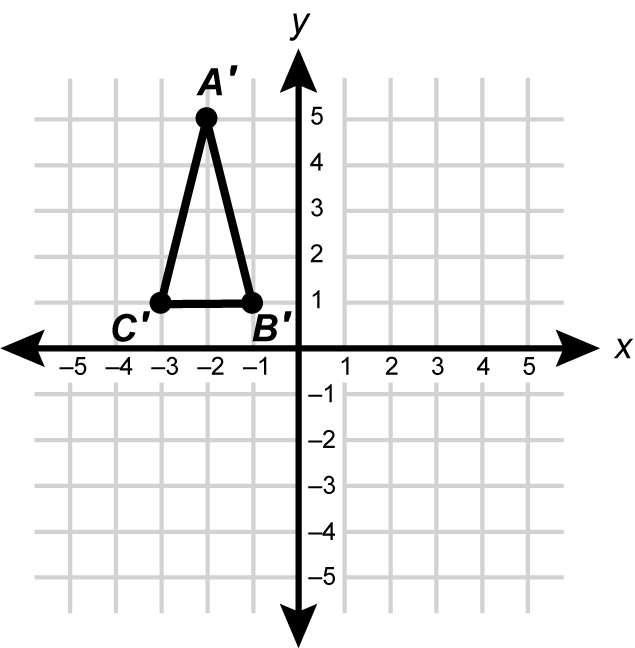
The image of triangle A B C is graphed in the coordinate plane with vertex A prime located at negative 2 5, vertex B prime located and negative 1 1 and vertex C prime located at negative 3 1.
-
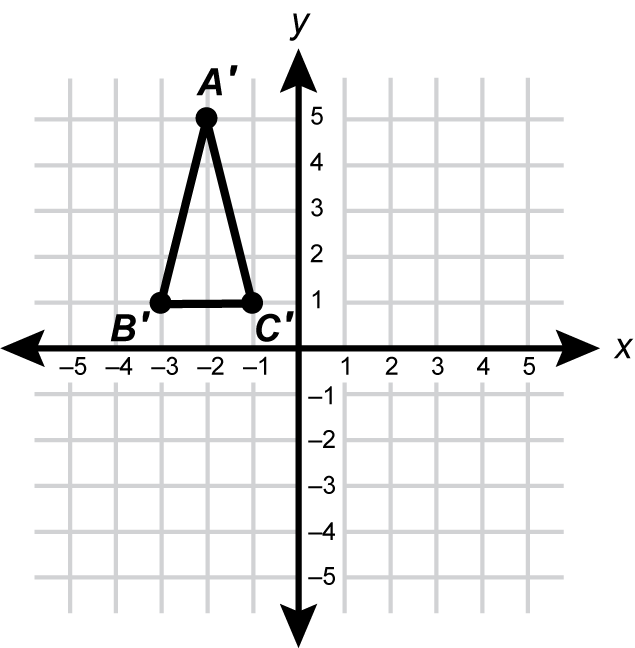
The image of triangle A B C is graphed in the coordinate plane with vertex A prime located at negative 2 5, vertex B prime located and negative 3 1 and vertex C prime located at negative 1 1.
-
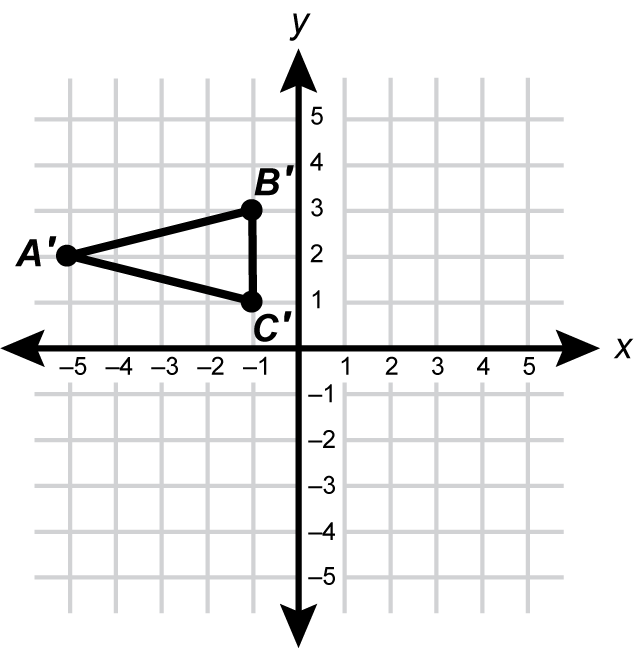
The image of triangle A B C is graphed in the coordinate plane with vertex A prime located at negative 5 2, vertex B prime located and negative 1 3 and vertex C prime located at negative 1 1.
-
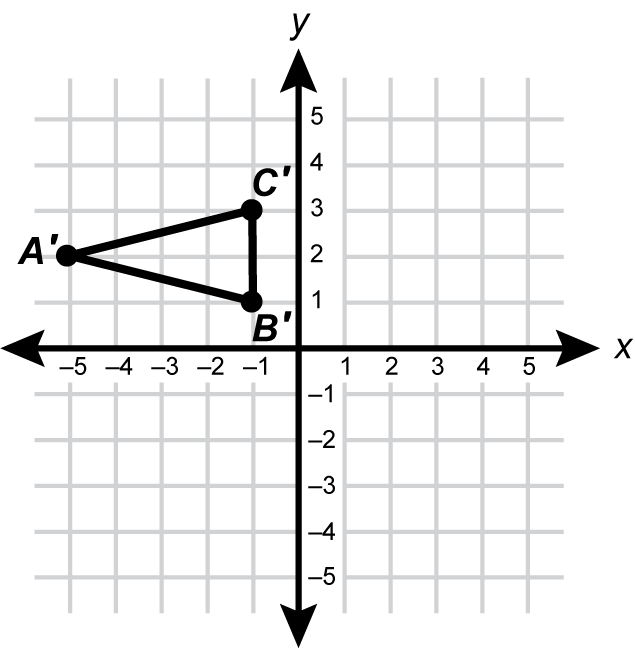
The image of triangle A B C is graphed in the coordinate plane with vertex A prime located at negative 5 2, vertex B prime located and negative 1 1 and vertex C prime located at negative 1 3.
Question 72.
Use the graph below to answer the question that follows.
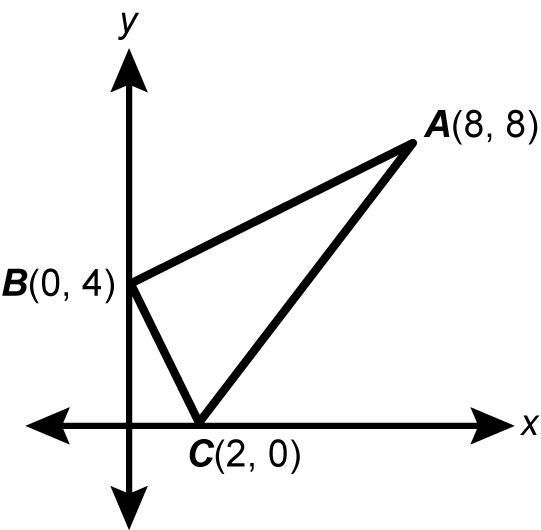
Triangle A B C is graphed in the first quadrant of a coordinate plane. Vertex A is located at 8 8, Vertex B is located at 0 4, and Vertex C is located at 2 0.
Which of the following expressions represents the area of the circle that circumscribes triangle A B C, given that A C is the diameter of the circle?
- 5 pi
- 10 pi
- 20 pi
- 25 pi
Question 73.
For any triangular region, the center of mass is located at the intersection of its three medians. If the center of mass of triangle A0, 0, B14, 0, C4, 12 is located at k, 4, what is the value of k?
- 8 thirds
- 10 thirds
- 6
- 7
Question 74.
Use the diagram below to answer the question that follows.
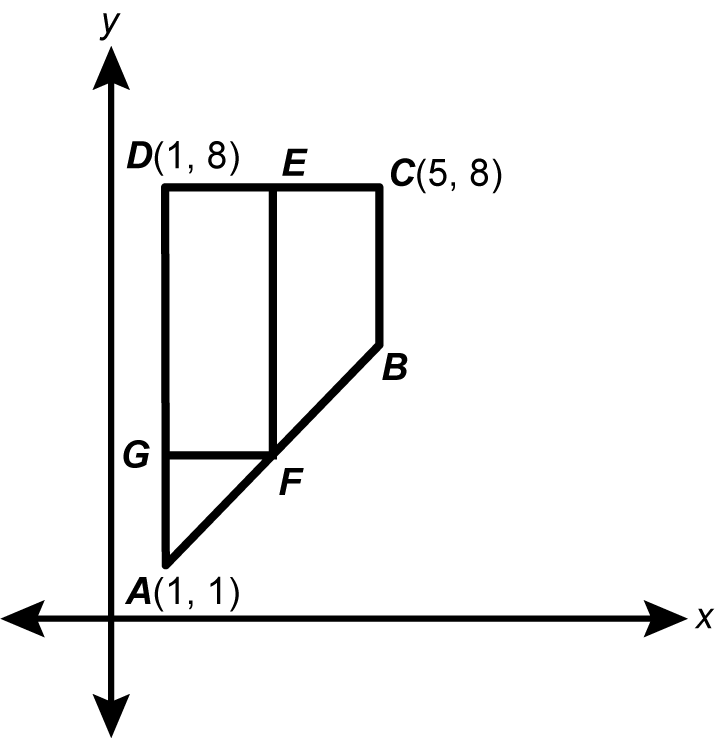
A trapezoid is shown in the first quadrant of the coordinate plane with vertex A at (1, 1), vertex B, vertex C at (5, 8), and vertex D located at (1, 8) There is a vertical line segment with endpoints E and F that is parallel to segment CB and bisects segment E C and segment A B. Another horizontal line segment connects point F to a point, G, located on segment A D.
Quadrilateral A B C D is constructed on a coordinate plane, as shown in the diagram. segment E F bisects segment D C and segment A B, and triangle A G F is isosceles. What is the area of the rectangle D E F G?
- 10
- 12
- 14
- 20
Question 75.
A bar graph would be the best choice for displaying which of the following sets of data?
- the percentage of total pieces of carry-on luggage that fall within various weight ranges for a particular airline
- the relationship between the number of hours a group of students spend reading and their scores on a standardized verbal skills test
- the number of pairs of sneakers of each of six brands sold in a season by a particular sporting goods store
- the average weekly earnings for construction workers over the last 20 years
Question 76.
Use the stem-and-leaf plot below to answer the question that follows.
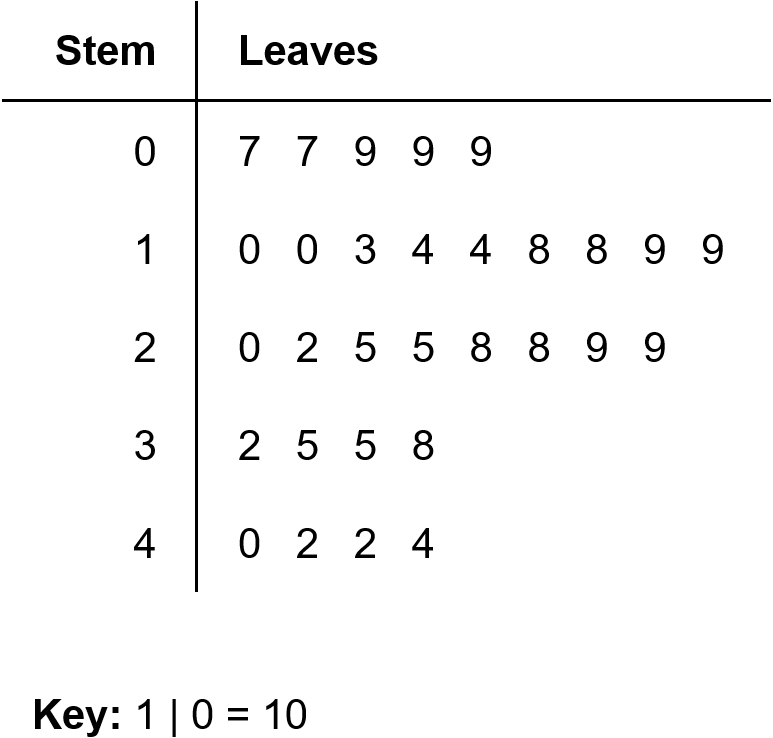
A stem and leaf plot is shown with stems 0, 1, 2, 3, and 4. Stem 0 has leaves 7 7 9 9 9. Stem 1 has leaves 0 0 3 4 4 8 8 9 9. Stem 2 has leaves 0 2 5 5 8 8 9 9. Stem 3 has leaves 2 5 5 8. Stem 4 has leaves 0 2 2 4. Key 1 to 0 equals 10
An athletic team plays ten games per season. The team's scores for the last three seasons are shown in the stem-and-leaf plot. What are the median score and interquartile range for these scores?
- median = 21, interquartile range = 19
- median = 23, interquartile range = 19
- median = 21, interquartile range = 37
- median = 23, interquartile range = 37
Question 77.
Use the data set below to answer the question that follows.
0, 0, 1, 1, 1, 2, 4, 4, 4, 4, 5, 6, 6, 7, 8
A human resources manager is compiling data on the number of sick days taken by employees during the previous year. The data are shown above. Which of the following statistics will be start bold least end bold affected if an employee who took 15 sick days is included in the data set?
- maximum
- mean
- median
- range
Question 78.
In a particular study, the heights of 50,000 16-year-old students were found to be normally distributed with a mean height of 168 centimeters and standard deviation 5 centimeters. Approximately how many students had a height of at least 173 centimeters?
- 8,000
- 16,000
- 34,000
- 42,000
Question 79.
A state representative tracks constituents' communications by phone, mail, or e-mail regarding a bill to be voted on. The representative counts 1500 total communications, with 70 percent in favor of the bill. Which of the following statements describes the validity of these results in representing the opinions of all constituents?
- The results must be valid because a large number of people made the effort to communicate with their representative.
- The results must be valid because all constituents have access to at least one of these methods of communication.
- The results may not be valid because a special interest group or groups may have been responsible for most of the favorable communications.
- The results may not be valid because the outcome of the vote for the bill is not yet known.
Question 80.
A student surveyed peers about two movies that were released over the weekend, a comedy movie and an animated movie. Of the peers the student surveyed, 55 percent of them had seen the comedy movie, 28 percent had seen the animated movie, and 14 percent had seen both movies. If the survey is representative of all students at the school, what is the probability that a randomly selected student saw neither movie?
- 13 percent
- 17 percent
- 31 percent
- 41 percent
Question 81.
Use the diagram below to answer the question that follows.
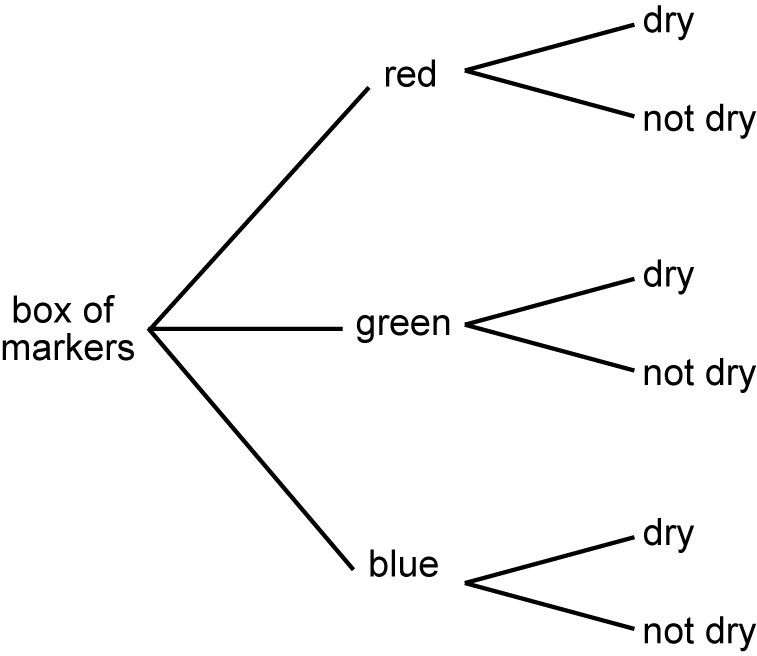
A diagram is shown with an initial point labeled "box of markers". Three lines branch out from the initial point labeled "red", "green", and "blue". At the end of each of those lines there are two branches labeled "dry" and "not dry" for a total of 6 branches at the end of the diagram.
The diagram above shows the composition of a box of used color markers in a classroom. There are 2 red markers, 4 green markers, and 6 blue markers. One-half of the red markers have dried out, 25 percent of the green markers are dry, and 50 percent of the blue markers are dry. What is the probability that a marker randomly selected from the box will be dried out?
- 3 twelfths
- 5 twelfths
- 6 twelfths
- 7 twelfths
Question 82.
A student places in a bag a blue number cube and two red number cubes, each with 6 faces numbered from 1 to 6. A friend will select two number cubes at random and then roll them. What is the probability that the result will be two red number cubes with a sum of 3?
- 1 fifty-fourth
- 1 thirty-sixth
- 1 twenty-second
- 1 eighteenth
Question 83.
In a bag containing only red and green grapes, there are 25 red grapes. After a child eats 5 green grapes, the probability of randomly choosing a green grape is less than 25 percent. What is the largest number of grapes that the bag could have contained originally?
- 43
- 39
- 38
- 31
Question 84.
A statistician is interested in determining the probability of getting at least one vowel (A, E, I, O, U) in random samples of the English alphabet. Which of the following simulations would generate data to answer this question?
- using a computer to randomly select 1000 five-letter words from a dictionary
- assigning the numbers 1through 26 to the letters Ato Z and using a calculator or computer to generate 100 random sets of five numbers
- designating each side of five coins as either vowel or consonant and flipping the set of five coins 100 times
- writing each letter of the alphabet on pieces of paper and randomly drawing a group of five-letter samples until a sample is drawn with only one vowel
Question 85.
Three red and three blue flags are arranged randomly along a wire. What is the probability that the six flags alternate in color?
- 1 twentieth
- 1 tenth
- 1 fourth
- 1 half
Open-Response Items
The directions shown below represent what you will see on the actual test. For the purposes of this practice test, you will be able to type your written responses in the boxes provided on the answer key.
This section of the test consists of two open-response item assignments. You will be asked to prepare a written response of approximately 150–300 words, or 1–2 pages, for each assignment.
Read the assignments carefully before you begin your responses. Think about how you will organize your responses. You may use the erasable sheet(s) to make notes, write an outline, or otherwise prepare your responses. However, your final response to each assignment must be either:
- typed into the on-screen response box,
- written on a response sheet and scanned using the scanner provided at your workstation, or
- provided using both the on-screen response box (for typed text) and a response sheet (for calculations or drawings) that you will scan using the scanner provided at your workstation.
Instructions for scanning your response sheet(s) are available by clicking the "Scanning Help" button at the top of the screen.
As a whole, your response to each assignment must demonstrate an understanding of the knowledge of the field. In your response to each assignment, you are expected to demonstrate the depth of your understanding of the subject area by applying your knowledge rather than by merely reciting factual information.
Your responses to the assignments will be evaluated based on the following criteria.
- Purpose: the extent to which the response achieves the purpose of the assignment
- Subject Knowledge: appropriateness and accuracy in the application of subject knowledge
- Support: quality and relevance of supporting evidence
- Rationale: soundness of argument and degree of understanding of the subject area
The open-response item assignments are intended to assess subject knowledge. Your responses must be communicated clearly enough to permit valid judgment of the evaluation criteria by scorers. Your responses should be written for an audience of educators in this field. The final version of each response should conform to the conventions of edited American English. Your responses should be your original work, written in your own words, and not copied or paraphrased from some other work.
Be sure to write about the assigned topics. Remember to review your work and make any changes you think will improve your responses.
Any time spent responding to an assignment, including scanning the response sheet(s), is part of your testing time. Monitor your time carefully. When your testing time expires, a pop-up message will appear on-screen indicating the conclusion of your test session. Only response sheets that are scanned before you end your test or before time has expired will be scored. Any response sheet that is not scanned before testing ends will NOT be scored.
Question 86.
Use the information below to complete the assignment that follows.
Standard
The Massachusetts Mathematics Curriculum Framework for grade 7 provides the following content standard:
Ratios and Proportions (7.RP)
3. Use proportional relationships to solve multi-step ratio, rate, and percent problems.
Problem Situation
A teacher leads students in a paper-folding activity. The students begin with a single sheet of paper. They observe that folding the paper in half once separates the sheet of paper into two sections. They also notice that folding the paper again doubles the number of boxes. The students are asked to record ratios that compare the number of times a paper is folded to the number of boxes that are formed. From these ratios, they are asked to predict how many folds will generate 128 boxes.
Assignment
Use your knowledge of mathematics to develop a response of approximately 150–300 words, or 1–2 pages, in which you analyze the number system with respect to content standard 7.RP.3. In your response:
- identify 2–3 related prerequisite concepts or skills, and explain their relevance to the mathematics described in the standard;
- create 2–3 appropriate representations (e.g., graphical, numerical, pictorial, verbal, physical) to model the mathematics described in the standard, and explain why each representation is appropriate; and
- critique whether the problem situation is aligned with the content standard in terms of the mathematics involved.
Be sure to show your work and explain the reasoning you use in analyzing the standard.
Question 87.
Use the information below to complete the assignment that follows.
A business is deciding whether to fulfill a customer's order for highly customized devices by using the services of their own manufacturing department or by arranging to have another manufacturer create the devices. The business will sell each device for $3500 regardless of who manufactures them.
In the process used by this business:
- 700 dollars worth of labor and materials is required to begin the first stage of manufacturing for each device. Upon completion of this stage, the device is tested for quality.
- The company expects that 80 percent of the devices created will meet quality standards and proceed to the second stage. The rest are discarded.
- An additional 500 dollars worth of labor and materials is required to begin the second stage of manufacturing for each device. Upon completion of this stage, the device is again tested for quality.
- The company expects that 75 percent of the devices that get to the second stage will meet quality standards and be sold to the client. The rest are discarded.
Use your knowledge of probability to develop a response of approximately 150–300 words, or 1–2 pages, in which you analyze the probabilities of specified outcomes. In your response:
- draw a tree diagram of the situation, including all appropriate labels, and use it to summarize the net profit or loss associated with every possible outcome in attempting to manufacture a single device;
- predict how many total manufacturing attempts will be needed to create 3 devices that can be sold;
- recommend whether the business should attempt to create the devices through their own manufacturing department or purchase 3 completed devices from another manufacturer for 9000 dollars total; and
- discuss any influences that can cause variations in your recommendation.
Be sure to show your work and explain the reasoning you use in analyzing and solving the problem.